Yaw Angles in Cycling: Part 2

January 3, 2017

[Editor's note: What follows is Part 2 of Nathan Barry's scholarly look at yaw angles experienced while cycling in triathlon, and their effect on the rider.]

In Part 1 we reviewed published data from several sources relating to the average yaw angles experienced by a cyclist. The results showed that low yaw angles are more dominant than high angles; generally following a typical bell curve or normal distribution. It was shown that we spend the majority of our time exposed to yaw angles less than 10deg, even on a notoriously windy course like Kona. However, this did not consider the effect of gusts on a cyclist which are also important to understand, especially the effect on handling.

Transient Winds

The average yaw distributions help describe the constant, or steady state, loads that a rider experiences. These are important for understanding drag performance, especially when translating wind tunnel data. However, a rider is also subject to gusts on top of the time-averaged cross wind, and it is these that we feel most strongly in the handling of our bike. As cyclists, we know what it feels like when exposed to a gust of wind, but we can describe that effect using physical models to better understand how this affects us.

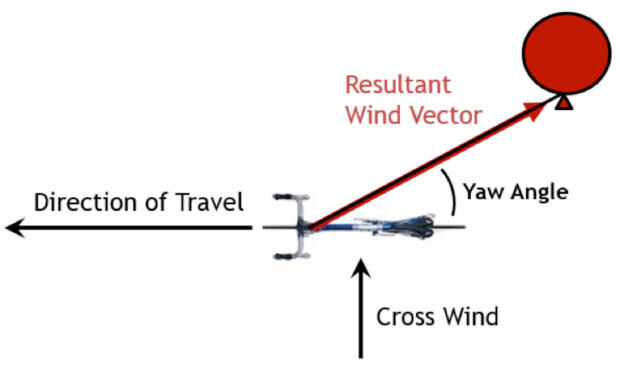
A gust of wind is effectively a spike in the yaw angle seen by a cyclist. Thinking back to the example of a balloon tied to the stem of your bike (as depicted below). If the cross wind component increases in velocity, even if orientation is unchanged, the effective yaw angle that results from the wind and road velocity vectors is larger. However, this is over a very short duration. In terms of aerodynamic loads, this can be modelled as a sudden shift laterally on the drag-yaw curve. Given a typical yaw curve this would equate to either a drag reduction (assuming an increase in yaw angle). If the gust is strong enough it is also possible to shift the yaw angle far enough to induce stall, such that drag starts to increase again. In the case of drag, these fluctuations are all in line with the direction of travel and therefore do not have a significant effect on handling. The loads that we feel most in gusts are side force and turning moments because they require control inputs to counteract. These can be modelled in a similar way by applying a lateral step on the yaw curve, provided we have data.

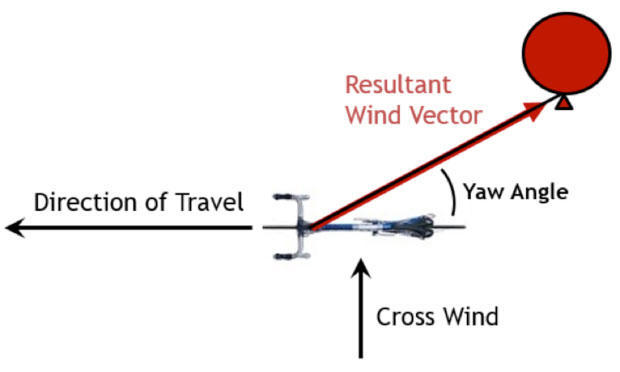
Handling in Cross Winds

Previously we discussed how the drag of a bicycle varies as a function of yaw angle. Whilst drag is the dominant form of resistance acting on a cyclist (under most conditions) it is not the only aerodynamic load. In fact there are six in total. 3 orthogonal forces on each axis of the bicycle and then 3 corresponding moments; one for each axis. Drag, side force, lift and then roll, pitch and yaw respectively (see image below). There is also a turning moment that acts on the front wheel about the steering axis.

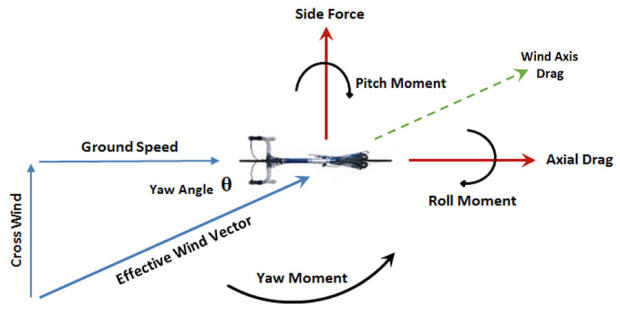
Drag, being a resistive force, directly opposes the rider’s motion. The other loads do not directly play into the performance equation (see Martin et al. 1998), however, we feel them in the handling of our bicycles.

Side force we experience as it acts to push the bike across the road. This requires a steering input to counteract. Whilst this results in scrubbing of the tyres, for the tyres used on road and triathlon bikes, the increased rolling resistance due to scrubbing is a small factor in total bicycle resistance.

Roll moment we experience as it acts to rotate the bike about the tyres contact point on the road; trying to tip the bike over. When riding we counteract this by leaning into the wind to generate an opposing moment. It is for this reason that taller and heavier riders are less susceptible to cross winds. Being taller and heavier results in a higher and larger centre of gravity. This results in a smaller angle of lean required to counteract the roll moment of the wind and higher inertia which resists gusts.

Steering torque is readily understandable from the perspective of a rider. If the centre of pressure for the front wheel and bars (the point of action for aerodynamic loads) is not located on the steering axis, then cross winds will act to rotate the wheel about the steering axis. The result is that the rider is required to provide a steering torque to oppose the wind load and maintain their line.

These actions are somewhat intuitive in learning to handle a bike. However, practice and concentration helps some riders to be more stable in cross winds. And whilst these loads do not directly act to slow a rider, it is apparent to anyone that has experienced extreme conditions of this type that such loads require concerted effort by the rider that can detract from their ability to ride at top speed and sap energy.

Wind axis vs Body axis?

The drag behaviour of a bicycle wheel, or even a bicycle, tested in isolation is characteristic of a wing or plate positioned at an angle of attack to the wind. This is largely also true when a rider is in place (see Barry et al. for discussion of differences). The key difference between wings and vehicles is the direction that we define drag. In the case of a plane or airfoil, drag is defined parallel to the direction of the wind (wind axis) whereas with a vehicle, drag is defined parallel to the vehicle’s direction of travel.

For an airfoil, as angle of attack is increased the resultant force vector increases. This is then decomposed into lift and drag. If we translate this scenario to a vehicle, as we increase yaw angle, the resultant vector is decomposed instead into a vehicle reference system for vehicle axis drag and side force. In this system a component of the resultant force can counteract the vehicle drag; much like a sailing boat generates thrust. This is why we typically see a decrease in drag as yaw angle increases for a bicycle or wheel. This occurs up to a point of minimum drag and then there is typically an inflection and drag increases beyond such a critical yaw angle. This occurs when the flow separates from the leeside surface(s). This is the same phenomenon that results in stall for an aircraft at high angles of attack. However, whilst drag typically decreases up to the stall point, side force increase with yaw angle.

Side force does not share the same characteristic behaviour as drag. Unlike a typical drag curve, side force does not have a stall point (see Barry et al., Greenwell). Side force is typically linear in its response, with a decreasing gradient at very extreme yaw angles. Therefore, under gust loads, the side force follows a more predictable response. The sudden change in load experienced by a rider is due to the changing yaw angle, and not the wheel or bike stalling.

Whilst the cycling public are becoming familiar with drag-yaw curves from wind tunnel tests, side force data is rarely published. Mostly because its effect on performance is not as direct as drag and more difficult to quantify, as described earlier. However, as we better understand the effects of side loads and moments on cycling dynamics, we can work towards reducing drag and, at the same time, minimising cross wind instability.

What does this mean for me?

The biggest take away for an athlete regarding cross winds is that you should have a personal understanding of how you handle and respond to cross winds. This means practising on your race wheels. Training on your deep wheels and race bike will let you get the feel for how your bike will respond on race day and you can improve your handling. This is especially important when riding on aerobars which offer less control than the wider grip of road handlebars. Treat it as a skill like any other aspect of bike riding; like cornering or dismounting.

Testing your wheels and setup in training will also help you to make better equipment choices as you learn your own boundaries. Some riders will be more confident in cross winds, due to anthropomorphic dimensions or skill level, and so what may be suitable for you may not work for all.

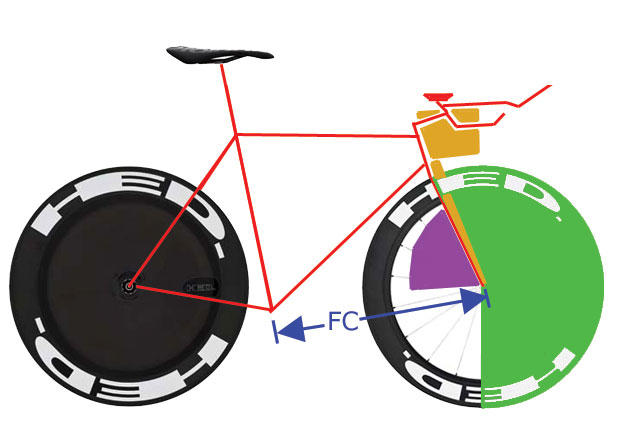
Take disc wheels as a good case in point. Because the disc wheel is on the back wheel it typically has less influence on handling than a front wheel. This is mostly due to the steering torque component. So you should not be scared to race it just because the wind is up. However, if you only ever ride a disc on still, flat courses, then you will only feel comfortable on still, flat roads. Practice with your race wheels and it will help you to reap the benefits of race day, even in strong winds.
Another take away is that you should be choosing your equipment based on aero performance at average yaw angles. As part 1 of this study showed, we are repeatedly seeing evidence that yaw angles experienced are predominantly in the +/- 10 degree range. This is not to say that high yaw performance should be ignored; you will still see high yaw angles at times. But these are very much secondary effects to performance at low yaw angles.

“Sailing a disc”

Some readers may have heard people talk about “sailing” a disk wheel. Whilst this may seem farfetched to those unfamiliar with sailing or aerodynamics, it is in fact a real effect. One key advantage of a disk wheel is that it acts as a wing to the wind. This can result in the resultant aerodynamic load counteracting drag. Much the way that a sail boat is able to move forwards using winds that are not aligned with the direction of travel. However, the trade-off is higher side force than spoked wheels. In this way, under cross wind conditions, a rider is effectively able to “sail” on their disk wheel. This effect is also present on deep section frame tubes and wheels but becomes most apparent for a disk wheel. For more detail on the effect of different types of wheels on aerodynamic loads see Barry et al. 2012.

Resources

Barry N, Burton D, Crouch T, Sheridan J and Luescher R, 2012, Effect of crosswind and wheel selection on the aerodynamic behaviour of a cyclist, Procedia Engineering, 34, p 20-25

Greenwell DI, Wood NJ, Bridge EKL and Addy RJ, 1995, Aerodynamic characteristics of low drag bicycle wheels, Aeronautical Journal, 99, p 109-120

Martin JC, Douglas ML, Cobb JE, McFadden KL and Coggan AR, 1998, Validation of a mathematical model for road cycling power, Journal of Applied Biomechanics, 14, p 276-291

About the author: Nathan Barry is a design engineer in the road group at Cannondale Bicycles. He has a PhD in cycling aerodynamics from Monash University in Australia and his research can be found in multiple peer-reviewed publications. Nathan has been racing triathlons for 15 years in everything from ITU to half ironman and off-road multisport.